No edit summary |
(update wp) |
||
| Line 1: | Line 1: | ||
{{BioPsy}} |
{{BioPsy}} |
||
| ⚫ | [[Image:Hodgkin-Huxley.jpg|thumb|right|350px|Basic Components of Hodgkin-Huxley type Models. Hodgkin-Huxley type models represent the biophysical characteristic of cell membranes. The lipid bilayer is represented as a capacitance (C<SUB>m</SUB>). Voltage-gated and leak ion channels are represented by nonlinear (g<SUB>n</SUB>) and linear (g<SUB>L</SUB>) conductances, respectively. The electrochemical gradients driving the flow of ions are represented by batteries (E), and ion pumps and exchangers are represented by current sources (I<SUB>p</SUB>).]] |
||
| − | The '''Hodgkin-Huxley Model''' is a set of non-linear ordinary differential equations, named after [[Alan Lloyd Hodgkin]] and [[Andrew Huxley]], that approximates the electrical characteristics of excitable cells such as neurons and cardiac myocytes. The original Hodgkin-Huxley model described the ionic mechanisms underlying the initiation and propagation of [[action potentials]] in the squid giant axon {{ref|HH}}. The model has played a seminal role in biophysics and neuronal modeling, but in 2006 was determined not to give an appropriate description for excitation transfer in [[cerebral cortex]] neurons of higher vertebrates {{ref|Naundorf}} as it is based on superseded assumptions of [[synapse]] function and were probably ''in vitro'' artifacts. For synapses in other contexts such as that from which the model was originally described, the Hodgkin-Huxley model is still considered valid pending further research. |
||
| + | The '''Hodgkin-Huxley Model''' is a [[scientific model]] that describes how [[action potential]]s in [[neuron]]s are initiated and propagated. |
||
| ⚫ | [[Image:Hodgkin-Huxley.jpg|thumb|right|Basic Components of Hodgkin-Huxley type Models. Hodgkin-Huxley type models represent the biophysical characteristic of cell membranes. The lipid bilayer is represented as a capacitance (C<SUB>m</SUB>). Voltage-gated and leak ion channels are represented by nonlinear (g<SUB>n</SUB>) and linear (g<SUB>L</SUB>) conductances, respectively. The electrochemical gradients driving the flow of ions are represented by batteries (E), and ion pumps and exchangers are represented by current sources (I<SUB>p</SUB>).]] |
||
| + | It is a set of [[nonlinearity|nonlinear]] [[ordinary differential equation]]s that approximates the electrical characteristics of excitable cells such as neurons and [[cardiac muscle|cardiac myocytes]]. |
||
| ⚫ | The components of a typical Hodgkin-Huxley model are shown in the figure. Each component of an excitable cell has a biophysical |
||
| + | |||
| + | [[Alan Lloyd Hodgkin]] and [[Andrew Huxley]] described the model in 1952 to explain the ionic mechanisms underlying the initiation and propagation of action potentials in the [[squid giant axon]].{{ref|HH}} They received the [[1963]] [[Nobel Prize in Physiology or Medicine]] for this work. |
||
| + | |||
| + | ==Basic Components== |
||
| + | |||
| ⚫ | The components of a typical Hodgkin-Huxley model are shown in the figure. Each component of an excitable cell has a biophysical analog. The [[lipid bilayer]] is represented as a [[capacitance]] (C<SUB>m</SUB>). [[Voltage-gated ion channel]]s are represented by [[nonlinear]] [[electrical conductance]]s (g<SUB>n</SUB>, where n is the specific ion channel), meaning that the conductance is voltage and time-dependent. This was later shown to be mediated by voltage-gated cation channel proteins, each of which has an open probability that is voltage-dependent. [[Leak channel]]s are represented by linear conductances (g<SUB>L</SUB>). The [[electrochemical gradient]]s driving the flow of ions are represented by batteries (E<SUB>n</SUB> and E<SUB>L</SUB>), the values of which are determined from the [[Nernst potential]] of the ionic species of interest. Finally, [[Ion pump (biology)|ion pumps]] are represented by [[current sources]] (I<SUB>p</SUB>). |
||
The time derivative of the potential across the membrane (<math>\dot{V}_m</math>) is proportional to the sum of the currents in the circuit. This is represented as follows: |
The time derivative of the potential across the membrane (<math>\dot{V}_m</math>) is proportional to the sum of the currents in the circuit. This is represented as follows: |
||
| Line 9: | Line 15: | ||
<math>\dot{V}_{m}= -\frac{1}{C_m} (\sum\limits ^{}_{i} I_{i} ),</math> |
<math>\dot{V}_{m}= -\frac{1}{C_m} (\sum\limits ^{}_{i} I_{i} ),</math> |
||
| − | where I< |
+ | where I<sub>i</sub> denotes the individual ionic currents of the model. |
| − | |||
| − | A reduced version of the Hodgkin-Huxley model was proposed by Richard FitzHugh and is now known |
||
| ⚫ | |||
==Ionic Current Characterization== |
==Ionic Current Characterization== |
||
The current flowing through the ion channels is mathematically represented by the following equation: |
The current flowing through the ion channels is mathematically represented by the following equation: |
||
| − | <math>{I}_{i}(V_m,t)= |
+ | <math>{I}_{i}(V_m,t)= (V_m - E_i) {g_i}\;</math> |
| + | |||
| + | where <math>E_i</math> is the [[reversal potential]] of the ''i''-th ion channel. |
||
In voltage-gated ion channels, the channel conductance g<SUB>i</SUB> is a function of both time and voltage (g<SUB>n</SUB>(t,V) in the figure), while in leak channels g<SUB>i</SUB> is a constant (g<SUB>L</SUB> in the figure). The current generated by ion pumps is dependent on the ionic species specific to that pump. The following sections will describe these formulations in more detail. |
In voltage-gated ion channels, the channel conductance g<SUB>i</SUB> is a function of both time and voltage (g<SUB>n</SUB>(t,V) in the figure), while in leak channels g<SUB>i</SUB> is a constant (g<SUB>L</SUB> in the figure). The current generated by ion pumps is dependent on the ionic species specific to that pump. The following sections will describe these formulations in more detail. |
||
| Line 32: | Line 37: | ||
where <math>\phi</math> and <math>\chi</math> are gating variables for activation and inactivation, respectively, representing the fraction of the maximum conductance available at any given time and voltage. <math>\bar{g}_n</math> is the maximal value of the conductance. <math>\alpha</math> and <math>\beta</math> are constants and <math>\tau_{\phi}</math> and <math>\tau_{\chi}</math> are the time constants for activation and inactivation, respectively. <math>\phi_{\infty}</math> and <math>\chi_{\infty}</math> are the steady state values for activation and inactivation, respectively, and are usually represented by Boltzmann equations as functions of <math>V_m</math>. |
where <math>\phi</math> and <math>\chi</math> are gating variables for activation and inactivation, respectively, representing the fraction of the maximum conductance available at any given time and voltage. <math>\bar{g}_n</math> is the maximal value of the conductance. <math>\alpha</math> and <math>\beta</math> are constants and <math>\tau_{\phi}</math> and <math>\tau_{\chi}</math> are the time constants for activation and inactivation, respectively. <math>\phi_{\infty}</math> and <math>\chi_{\infty}</math> are the steady state values for activation and inactivation, respectively, and are usually represented by Boltzmann equations as functions of <math>V_m</math>. |
||
| − | In order to characterize voltage-gated channels, the equations will be fit to voltage-clamp data. For a derivation of the Hodgkin-Huxley equations under voltage-clamp see |
+ | In order to characterize voltage-gated channels, the equations will be fit to voltage-clamp data. For a derivation of the Hodgkin-Huxley equations under voltage-clamp see.{{ref|JohnstonAndWu}} Briefly, when the membrane potential is held at a constant value (i.e., voltage-clamp), for each value of the membrane potential the nonlinear gating equations reduce to linear differential equations of the form: |
<math>\phi(t) = \phi_{0} - [ (\phi_{0}-\phi_{\infty})(1 - e^{-t/\tau_{\phi}})] </math> |
<math>\phi(t) = \phi_{0} - [ (\phi_{0}-\phi_{\infty})(1 - e^{-t/\tau_{\phi}})] </math> |
||
| Line 42: | Line 47: | ||
<math>I_{n}(t)=\bar{g}_{n} \phi^{\alpha} \chi^{\beta} (V_{m}-E_{n})</math>. |
<math>I_{n}(t)=\bar{g}_{n} \phi^{\alpha} \chi^{\beta} (V_{m}-E_{n})</math>. |
||
| − | The [[Levenberg-Marquardt algorithm]] |
+ | The [[Levenberg-Marquardt algorithm]],{{ref|Marquardt}}{{ref|Levenberg}} a modified [[Gauss-Newton algorithm]], is often used to fit these equations to voltage-clamp data. |
===Leak Channels=== |
===Leak Channels=== |
||
| Line 48: | Line 53: | ||
===Pumps and Exchangers=== |
===Pumps and Exchangers=== |
||
| − | The membrane potential depends upon the maintenance of ionic concentration gradients across it. The maintenance of these concentration gradients requires active transport of ionic species. The sodium-potassium and sodium-calcium exchangers are the best known of these. Some of the basic properties of the Na/Ca exchanger have already been well-established: the stoichiometry of exchange is 3 Na<SUP>+</SUP>:1 Ca<SUP>2+</SUP> and the exchanger is electrogenic and voltage-sensitive. The Na/K exchanger has also been described in detail |
+ | The membrane potential depends upon the maintenance of ionic concentration gradients across it. The maintenance of these concentration gradients requires active transport of ionic species. The sodium-potassium and sodium-calcium exchangers are the best known of these. Some of the basic properties of the Na/Ca exchanger have already been well-established: the stoichiometry of exchange is 3 Na<SUP>+</SUP>:1 Ca<SUP>2+</SUP> and the exchanger is electrogenic and voltage-sensitive. The Na/K exchanger has also been described in detail.{{ref|Hille}} |
| + | |||
| + | ==See also== |
||
| ⚫ | |||
| + | *[[Soliton model]] |
||
| + | *[[Action potential]] |
||
| + | *[[Biological neural network]] |
||
==References== |
==References== |
||
#{{note|HH}}Hodgkin, A., and Huxley, A. (1952): A quantitative description of membrane current and its application to conduction and excitation in nerve. ''J. Physiol.'' '''117''':500–544. PMID 12991237 |
#{{note|HH}}Hodgkin, A., and Huxley, A. (1952): A quantitative description of membrane current and its application to conduction and excitation in nerve. ''J. Physiol.'' '''117''':500–544. PMID 12991237 |
||
| − | #{{note|Naundorf}}Naundorf, B. ''et al.'' (2006): Unique features of action potential initiation in cortical neurons. ''[[Nature (journal)|Nature]]'' '''440''' (7087): 1060-1063. [[Digital Object Identifier|DOI]]:10.1038/nature04610 [http://dx.doi.org/10.1038/nature04610 HTML abstract] [http://www.nature.com/nature/journal/v440/n7087/suppinfo/nature04610.html Supplementary information] |
||
#{{note|Marquardt}}Marquardt, D. (1963): An algorithm for the least-squares estimation of nonlinear parameters. ''SIAM J. Appl. Math.'' '''11''' (2):431–441. |
#{{note|Marquardt}}Marquardt, D. (1963): An algorithm for the least-squares estimation of nonlinear parameters. ''SIAM J. Appl. Math.'' '''11''' (2):431–441. |
||
#{{note|Levenberg}}Levenberg, K. (1944): A method for the solution of certain non-linear problems in least-squares. ''Q. Appl. Math.'' '''2''' (2):164–168. |
#{{note|Levenberg}}Levenberg, K. (1944): A method for the solution of certain non-linear problems in least-squares. ''Q. Appl. Math.'' '''2''' (2):164–168. |
||
| − | #{{note|JohnstonAndWu}}Johnston, D., and Wu, S. (1997): Foundations of Cellular Neurophysiology, chapter |
+ | #{{note|JohnstonAndWu}}Johnston, D., and Wu, S. (1997): Foundations of Cellular Neurophysiology, chapter 6. MIT Press, Cambridge, MA. ISBN 0-262-10053-3 |
#{{note|Hille}}Hille, B. (2001): Ionic Channels of Excitable Membranes (3rd ed.). Sinauer Associates, Inc., Sunderland, MA. ISBN 0-87893-321-2 |
#{{note|Hille}}Hille, B. (2001): Ionic Channels of Excitable Membranes (3rd ed.). Sinauer Associates, Inc., Sunderland, MA. ISBN 0-87893-321-2 |
||
| + | |||
| + | ==External links== |
||
| + | *[http://thevirtualheart.org/HHindex.html Interactive Java applet of the HH model ] Parameters of the model can be changed as well as excitation parameters and phase space plottings of all the variables is possible. |
||
| + | *[http://comp.uark.edu/~jostmey/Hodgkin-Huxley%20Equations/Hodgkin-Huxley%20Applet.html Java applet of the HH Equations] Numerically solves the Hodgkin-Huxley Equations. Parameters may be varied, and allows for user to select from any arbitrary current. |
||
[[Category:Non-linear systems]] |
[[Category:Non-linear systems]] |
||
| Line 62: | Line 76: | ||
[[Category:Ion channels]] |
[[Category:Ion channels]] |
||
[[Category:Computational neuroscience]] |
[[Category:Computational neuroscience]] |
||
| + | [[Category:Excitable Membranes]] |
||
| − | + | :de:Hodgkin-Huxley-Modell |
|
| + | :he:מודל הודג'קין-הקסלי |
||
{{enWP|Hodgkin-Huxley model}} |
{{enWP|Hodgkin-Huxley model}} |
||
Latest revision as of 17:03, 9 December 2007
Assessment |
Biopsychology |
Comparative |
Cognitive |
Developmental |
Language |
Individual differences |
Personality |
Philosophy |
Social |
Methods |
Statistics |
Clinical |
Educational |
Industrial |
Professional items |
World psychology |
Biological: Behavioural genetics · Evolutionary psychology · Neuroanatomy · Neurochemistry · Neuroendocrinology · Neuroscience · Psychoneuroimmunology · Physiological Psychology · Psychopharmacology (Index, Outline)
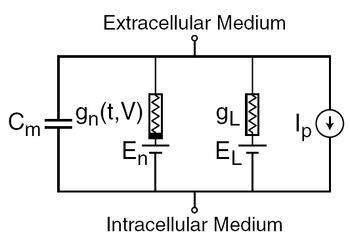
Basic Components of Hodgkin-Huxley type Models. Hodgkin-Huxley type models represent the biophysical characteristic of cell membranes. The lipid bilayer is represented as a capacitance (Cm). Voltage-gated and leak ion channels are represented by nonlinear (gn) and linear (gL) conductances, respectively. The electrochemical gradients driving the flow of ions are represented by batteries (E), and ion pumps and exchangers are represented by current sources (Ip).
The Hodgkin-Huxley Model is a scientific model that describes how action potentials in neurons are initiated and propagated. It is a set of nonlinear ordinary differential equations that approximates the electrical characteristics of excitable cells such as neurons and cardiac myocytes.
Alan Lloyd Hodgkin and Andrew Huxley described the model in 1952 to explain the ionic mechanisms underlying the initiation and propagation of action potentials in the squid giant axon.[1] They received the 1963 Nobel Prize in Physiology or Medicine for this work.
Basic Components
The components of a typical Hodgkin-Huxley model are shown in the figure. Each component of an excitable cell has a biophysical analog. The lipid bilayer is represented as a capacitance (Cm). Voltage-gated ion channels are represented by nonlinear electrical conductances (gn, where n is the specific ion channel), meaning that the conductance is voltage and time-dependent. This was later shown to be mediated by voltage-gated cation channel proteins, each of which has an open probability that is voltage-dependent. Leak channels are represented by linear conductances (gL). The electrochemical gradients driving the flow of ions are represented by batteries (En and EL), the values of which are determined from the Nernst potential of the ionic species of interest. Finally, ion pumps are represented by current sources (Ip).
The time derivative of the potential across the membrane () is proportional to the sum of the currents in the circuit. This is represented as follows:
where Ii denotes the individual ionic currents of the model.
Ionic Current Characterization
The current flowing through the ion channels is mathematically represented by the following equation:
where is the reversal potential of the i-th ion channel.
In voltage-gated ion channels, the channel conductance gi is a function of both time and voltage (gn(t,V) in the figure), while in leak channels gi is a constant (gL in the figure). The current generated by ion pumps is dependent on the ionic species specific to that pump. The following sections will describe these formulations in more detail.
Voltage-Gated Ion Channels
Under the Hodgkin-Huxley formulation, conductances for voltage-gated channels (gn(t,V)) are expressed as:
,
where and are gating variables for activation and inactivation, respectively, representing the fraction of the maximum conductance available at any given time and voltage. is the maximal value of the conductance. and are constants and and are the time constants for activation and inactivation, respectively. and are the steady state values for activation and inactivation, respectively, and are usually represented by Boltzmann equations as functions of .
In order to characterize voltage-gated channels, the equations will be fit to voltage-clamp data. For a derivation of the Hodgkin-Huxley equations under voltage-clamp see.[2] Briefly, when the membrane potential is held at a constant value (i.e., voltage-clamp), for each value of the membrane potential the nonlinear gating equations reduce to linear differential equations of the form:
Thus, for every value of membrane potential, , the following equation can be fit to the current curve:
.
The Levenberg-Marquardt algorithm,[3][4] a modified Gauss-Newton algorithm, is often used to fit these equations to voltage-clamp data.
Leak Channels
Leak channels account for the natural permeability of the membrane to ions and take the form of the equation for voltage-gated channels, where the conductance is a constant.
Pumps and Exchangers
The membrane potential depends upon the maintenance of ionic concentration gradients across it. The maintenance of these concentration gradients requires active transport of ionic species. The sodium-potassium and sodium-calcium exchangers are the best known of these. Some of the basic properties of the Na/Ca exchanger have already been well-established: the stoichiometry of exchange is 3 Na+:1 Ca2+ and the exchanger is electrogenic and voltage-sensitive. The Na/K exchanger has also been described in detail.[5]
See also
- Fitzhugh-Nagumo model
- Soliton model
- Action potential
- Biological neural network
References
- ^ Hodgkin, A., and Huxley, A. (1952): A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 117:500–544. PMID 12991237
- ^ Marquardt, D. (1963): An algorithm for the least-squares estimation of nonlinear parameters. SIAM J. Appl. Math. 11 (2):431–441.
- ^ Levenberg, K. (1944): A method for the solution of certain non-linear problems in least-squares. Q. Appl. Math. 2 (2):164–168.
- ^ Johnston, D., and Wu, S. (1997): Foundations of Cellular Neurophysiology, chapter 6. MIT Press, Cambridge, MA. ISBN 0-262-10053-3
- ^ Hille, B. (2001): Ionic Channels of Excitable Membranes (3rd ed.). Sinauer Associates, Inc., Sunderland, MA. ISBN 0-87893-321-2
External links
- Interactive Java applet of the HH model Parameters of the model can be changed as well as excitation parameters and phase space plottings of all the variables is possible.
- Java applet of the HH Equations Numerically solves the Hodgkin-Huxley Equations. Parameters may be varied, and allows for user to select from any arbitrary current.
- de:Hodgkin-Huxley-Modell
- he:מודל הודג'קין-הקסלי
| This page uses Creative Commons Licensed content from Wikipedia (view authors). |

















![{\displaystyle \phi (t)=\phi _{0}-[(\phi _{0}-\phi _{\infty })(1-e^{-t/\tau _{\phi }})]}](https://services.fandom.com/mathoid-facade/v1/media/math/render/svg/951b3681d2a9af1dd7bcad9e89328a36f4d80bbb)
![{\displaystyle \chi (t)=\chi _{0}-[(\chi _{0}-\chi _{\infty })(1-e^{-t/\tau _{\chi }})]}](https://services.fandom.com/mathoid-facade/v1/media/math/render/svg/10f982733fa1c3ec6a816854a6d2cef8433b80ba)

